Expanded as a series, the exponential factor describing the x-ray radiation field at the absorbing atom or molecule has the form

Within the dipole approximation, the differential cross section (cross section per unit solid angle) for angle-resolved photoemission with linearly polarized x rays is described by three quantities: the partial cross section, s(hn), the angular-distribution parameter, b(hn), and the angle (q) of the photoelectron trajectory relative to the polarization vector. When extracted from angular distribution measurements, s(hn) and b(hn) provide information about the electronic structure of the atom and the molecule and the dynamics of the photoionization process. For example, at the "magic angle" q = 54.7º, the angular term disappears and s(hn) is obtained.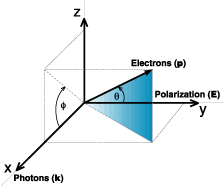

In the dipole approximation, a single term describes electron angular distributions as a function of the angle q relative to the polarization, E, of the radiation. Higher-order photon interactions lead to nondipole effects, which in the experiments reported here can be described by two new parameters and a second angle, f, relative to the propagation direction, k, of the radiation.

Finding the Devil in the Details

Scientists studying atoms and molecules use x rays to determine the "electronic structure" comprising the electron orbitals and their characteristics. Photoemission is a good example. From the spectrum of kinetic energies and directions of travel of photoelectrons emitted from the atom or molecule after absorbing x rays, investigators can work backwards to reconstruct the electronic structure. However, this process requires theoretical models that not only accurately describe the interaction of the x ray with the atom or molecule but that are also solvable in a practical way.

For this reason, scientists use as much as possible a simplification of the x-ray interaction called the dipole approximation. However, in the last few years, scientists conducting very careful measurements at the ALS and elsewhere have found that in surprising circumstances the dipole approximation is not sufficiently accurate. A more accurate approximation with extra "first-order" corrections helped but did not eliminate the discrepancies between theory and experiment in every case. Now a collaboration of theorists and experimentalists working at the ALS has both calculated the effects of even more sophisticated "second-order" corrections and experimentally verified their importance at unexpectedly low energies. Researchers in several fields may now need to take these nondipole effects into account.

It has long been known that this approximation is not valid for high photon energies (e.g., above 5 keV), where the photon wavelength is smaller than the size of the atom or molecule. In the last few years, groups working at the ALS and elsewhere have shown that additional first-order nondipole (specifically, electric quadrupole) terms are needed in the rare gases at lower photon energies and close to an ionization threshold. These terms involve two first-order energy-dependent parameters, d(hn) and g(hn), and a new angle variable (f).

At this level of approximation, the recent rare-gas experiments showed significant modifications of the photoelectron angular distributions compared to those expected within the dipole approximation, modifications that were in generally good agreement with the first-order calculations. However, when conducting the analysis for neon in terms of the g(hn) for 2s photoemission and z(hn) (where z = 3d + g) for 2p photoemission, experimenters at the ALS noticed that some discrepancy remained, particularly for neon 2p photoemission.

Theorists among the group calculated a general expression for the angle-resolved photoemission cross section including second-order contributions, which introduced four new energy-dependent nondipole factors dominated by electric-octupole and pure electric-quadrupole effects. Since no new angles were involved, the second-order corrections could then be recast in terms of effective values of g(hn) and z(hn) for comparison with their measurements on neon.

The group made this comparison for four geometries, two with q at the magic angle where only nondipole terms are important, and two on a "nondipole cone" at an angle of 35.3º around the direction of the x-ray beam. Comparison of experiment with first-order theory yielded good agreement for both neon 2s and 2p photoemission for detectors on the nondipole cone, but in the magic-angle geometry, second-order corrections were needed, especially for neon 2p.

Experimental and theoretical values of the first-order correction terms g2s and z2p for neon 2s and 2p photoemission determined in "magic-angle" and "nondipole-cone" geometries.

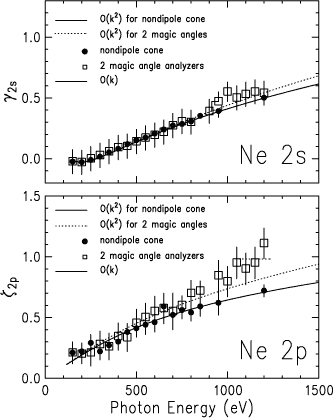
The complex angular dependence of the differential cross section means that which corrections to the dipole approximation are needed depends on the experimental geometry, but the new results demonstrate that researchers need to be ready to include nondipole effects through at least the second order in analyzing their results.

Research conducted by A. Derevianko and W.R. Johnson (University of Notre Dame); O. Hemmers, S. Oblad, and D.W. Lindle (University of Nevada, Las Vegas); P. Glans (Stockholm University); H. Wang (Uppsala University); S.B. Whitfield (University of Wisconsin); R. Wehlitz (University of Wisconsin); and I.A. Sellin (University of Tennessee, Knoxville).

Research funding: National Science Foundation, EPSCoR Program of the U.S. Department of Energy, and University of Nevada, Las Vegas. Operation of the ALS is supported by the Office of Basic Energy Sciences, U.S. Department of Energy.

Publication about this experiment: A. Derevianko, O. Hemmers, S. Oblad, P. Glans, H. Wang, S.B.Whitfield, R. Wehlitz, I.A. Sellin, W.R. Johnson, and D.W. Lindle, "Electric-Octupole and Pure-Electric-Quadrupole Effects in Soft-X-Ray Photoemission," Phys. Rev. Lett. 84, 2116 (2000).

ALSNews Vol. 170, February 14, 2001